The Literacy of Numeracy Part 2: Explicit Vocabulary Instruction

Mr Morgs’ follow up blog on how children can approach the language used in the reasoning papers in the KS2 SATs.

This is the 2nd part of my ‘literacy of numeracy’ blogpost series. The first analysed the language used in SATs tests and suggested tips you can use in the classroom to help prepare children for the tests based off of the analysis. You can find part 1 here – http://nexus-education.com/ks2-maths-sats/http://nexus-education.com/ks2-maths-sats/ and a free downloadable version with a PowerPoint you can use in class here – https://www.tes.com/teaching-resource/ks2-maths-sats-language-analysis-12076440

I originally delivered this current blog as a 30-minute speech at an education conference and have tried my best to trim it down.

Introduction

In this blog series, I have been titling the posts under the umbrella phrase – ‘the literacy of numeracy’. I want to begin by clarifying what I mean by that. This phrase concerns itself with the disciplinary literacy of mathematics. Put more simply, ensuring each child is numerically literate by teaching them how to interpret visual symbols, graphs, charts, diagrams as well as individual terms and word problems. I believe language is the principle factor in developing this literacy within children and that the teaching of reasoning and problem solving in its current form can be very ineffective, when it relies on basic strategies like the use of RUCSAC and circling and underlining keywords.

Following a more language-specific method can prove to be more fruitful, as mathematics presents a lot of issues through its frequent variety of representation (e.g. 500g, 0.5kg and 1/2kg), but often the language used remains consistent. Maths can be considered as an additional language, in which it has its own specific terms and syntax. Enumerated below are just a few language obstacles children face in our classrooms:

1. Their knowledge of synonyms usually linked to one of the four calculation types (take instead of subtract, product instead of answer, altogether rather than add)
2. Their understanding of superlatives (biggest, largest, tallest, smallest)
3. Words that can have different meanings outside of a mathematical context (round, product, factor, prime)
4. Words other than superlatives that suggest comparison (between, more/less than, each, share, in order, sorting, put in the correct place)
5. Their understanding of the difference between the right answer and the wrong answer (best estimate, explain why Jack is not correct, write the correct symbol in each box, circle the improper fraction that is equivalent)
6. Verbs implying mathematical meaning (remaining, left, combine, collect, spend)

7. Compression of vocabulary through nominalisation and noun phrases – prime number, improper fraction, roman numeral, perpendicular and parallel lines, 3D shape
8. Abstract nouns – circumference, multiplication, area, perimeter

I emphasise this idea of maths as its own language, because as a maths SATs marker this year, I saw countless children incorrectly answer Reasoning Paper 2 Q18 (pictured below) because they indicated that ’95 goes into 5′ and ’87 goes into 3′. In my two years of SATs marking, I have seen far too many marks lost to inarticulation. Interestingly, in the 1068 responses to Paper 2 Q18 I marked, I only saw 1 child use the term ‘composite’. Naturally, you did not need to use this term to explain the answer correctly but I thought it was indicative of a wider issue: the lack of explicit vocabulary teaching in mathematics. Somebody once said that, “you shall know a word by the company it keeps”. While this may be true in fictional texts, these context clues aren’t always as evident in the world of maths. While normal vocabulary instruction often permits the use of analogy or synonym, the opposite is true in teaching the vocabulary of maths – where a singular definition is required and needs to be precise. Naturally, synonyms are still rife in maths (e.g. multiply, times), but the focus must be on teaching specific maths vocabulary, in order for children to be able to reason and problem solve freely without difficulty, especially as the style of language and vocabulary used in the non-fiction texts of the maths classroom are different in nature to the fiction texts children usually read.

Making it Accessible for All
As should be our intent for all our teaching, we must make the learning accessible for all in the classroom: our use of language is how we achieve this. Typically, the language that children read is more difficult than the language we use in our interactions with them. Therefore, there are two effects I believe to be present in the classroom. Firstly, the Dunning-Kruger effect, where children think they understand, but are unable to accurately communicate their thoughts and reasoning. Secondly, we as teachers suffer from the ‘curse of knowledge’, because we are experts transferring knowledge to novices, and we naturally overestimate their background knowledge and starting points. A consistent approach based around keywords is needed to combat both of these effects successfully. After all, the underlying fabric of maths teaching is to develop cognitive growth and the ability to reason, not to simply stack pieces of knowledge on top of each other.
Are the children that Alex Quigley defines as ‘word poor’ ever able to achieve mastery or greater depth? Language is an inevitable obstacle for them. This can be in part attributed to the fact that too much academic vocabulary remains implicit (e.g. explain, estimate, identify). These words require explicit vocabulary instruction, like any other word being learnt in any other subject. The language of the classroom and our everyday language are intertwined and should be more distinctly separate. For example, we may say that ‘we take from here and put it there’ rather than ‘we transfer from the hundreds column into the tens column’. We cannot successfully construct and convey the meaning of mathematics if we rely so heavily on language from everyday usage. The use of keywords is a simple way to combat this, as it allows for a manageable consistency; understanding can then be mapped and revisited more easily and it makes retrieval far simpler. As a year 6 teacher, I always thought about this approach when considering the transition to secondary school. The use of keywords in specific topics is likely to be consistent between our lessons in primary and secondary, whereas the use of individual and personal colloquialisms will not necessarily pervade.
Implementation
For me, this approach was born out of necessity. Working memory was becoming worse with each new class and the SATs were becoming increasingly hard following the introduction of the new curriculum – there seemed to be an increased demand on retrieval ability and the old level 6 questions were now arguably integrated at the back of the reasoning papers. I wanted to create an approach that was easy for teachers to remember and easy for them to implement. I ended up with two simple questions that we wanted the children to ask themselves:

• What does the question want us to find out? (i.e. how many litres left, a fraction of the shape etc)
• What do we have to do in order to find that answer? (i.e. subtract from the total, change to equivalent fractions etc)

The idea of these two questions is to blur the traditional gap between the words on the page (questions) and the words in our head (thought process) and we can achieve this through explicit vocabulary instruction. Research shows that children who are exposed to explicit vocabulary teaching benefit 3 times more than those who are not. In that sense, reasoning and problem solving activities must be treated as their own genre of reading that requires comprehensions skills taught around it (see part one of the blog for more on that). In order to do so, we must place an increased focus on both academic (e.g. estimate, identify) and subject-specific (e.g. numerator, multiple) vocabulary, like we do so readily in other subjects.
Children from Year 2 up can be taught to write down their reasoning justifications. Within these, they should be encouraged to use keywords to demonstrate their knowledge of them and how they exist within their own mathematical concepts. This should be done frequently, with at least one opportunity for it every lesson (e.g. a starter or plenary, responding to a further learning question from your marking).
In assessing children’s knowledge of keywords, Cronbach’s (1942) dimensions of word knowledge is a useful guide. It is also very informative as to how we should plan and deliver our lessons:

1. Generalisation – the ability to define a word
2. Application – the ability to apply the word in appropriate situations
3. Breadth – the ability to know and recall different meanings of the word
4. Precision – the ability to recognise exactly in what situations the word does and does not apply
5. Availability – the ability to use the word in our thinking and in our speech

Here is a basic example of a child in my class using the keywords they have been taught to confidently explain their reasoning (isosceles):

‘We know isosceles has two sides the same length which means they are equally spaced out. And the difference in the y is (6,8) (32, 18) so we have to add another 10 to the y which is 28. The whole answer is (6, 28).
Children need to be provided with the opportunity to reason frequently and our lessons can allow this through both verbal (answering questions aloud, discussions etc) and written opportunities (writing down their reasoning, independently attempting problems etc). The former being more important lower down the school (EYFS, Year 1), as younger children’s listening and speaking skills are superior to their written and reading skills. But no matter how good the opportunities we provide are, the only way this can be truly successful is for us to have the ability to improvise and respond effectively to whatever children say to us. That sounds easier said than done, and it is, but I believe as long as we have a depth of subject knowledge and are well aware of the common misconceptions children have, then we can improvise fairly effectively.
When it comes to introducing and teaching the definitions of words, I have found it effective to change definitions regularly so that children weren’t reliant on memorising a single definition and had to constantly access prior knowledge each time a new definition was formulated – for example, this could include blacking out words in a definition or providing different accompany examples to ones they have previously seen. But definitions aren’t enough. We need rich instruction. Here are just a few examples of things we can do to provide this alongside our definitions:

• use of pictorial representation
• etymology
• morphology
• root words (vertere = to turn)
• links to other words and concepts (vertex, inverse, convert and vertical – what is the link?)
• examples and non-examples
• keywords in lesson objectives (and discussing these LOs)
• frequent reasoning opportunities that use keywords and encourage children to use the keywords

Further comments about the use of keywords:

• We should employ a specific focus on visual representation alongside them, as often a child’s lack of vocabulary can hinder them in understanding new words being taught to them and it can make it hard for them to break down even the simplest of definitions. It is often these children that argue they ‘cannot do maths’ when simply they are unable to use mathematical language to explain their thoughts.
• Discussion of keywords is of the utmost importance because we must create an atmosphere where all answers want to be heard – right or wrong. We cannot develop understanding and take children to the next level without knowing if they misunderstand first.
• We must make the work accessible for the children who struggle more – do not give a year 6 child year 4 work. Give them year 6 work that is pitched at their level (more pictures, breakdown of keywords used, methods explicitly stated alongside keywords etc).
• If the meaning of keywords is only ever presented by the teacher and not negotiated with the class, then children will never consciously construct meaning for themselves. Meaning is derived from the context within which the language is being used, and the dialogue between teacher and child is what frames this.

Here is a picture of a slide that I might typically show at the start of a lesson to my class. It includes a pictorial representation of the definition as an example, the root word and a part blacked out to challenge their thought on what the definition is:

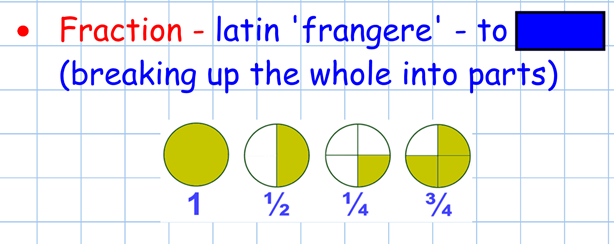
I’ll end this blog with Charles Darwin’s very apt description of mathematics – “A mathematician is a blind man in a dark room looking for a black cat which isn’t there”. Language is the light that can help to cure the blindness, illuminate the room and put the black cat right in your hands.
I plan on carrying on this series by writing about mastery in maths and what a perfect lesson might look like, with reference to what has been said above.
Contact me on Twitter if you have any questions – @_mrmorgs

The author

Mr Morgs

Mr Morgs is an experienced Year 6 teacher from South London. He is a trained SATs marker, LA Moderator and has an MA in leadership. He is interested in keeping up-to-date with current educational research, specifically related to the teaching of maths and reading.