


Our team sorts through all blog submissions to place them in the categories they fit the most - meaning it's never been simpler to gain advice and new knowledge for topics most important for you. This is why we have created this straight-forward guide to help you navigate our system.
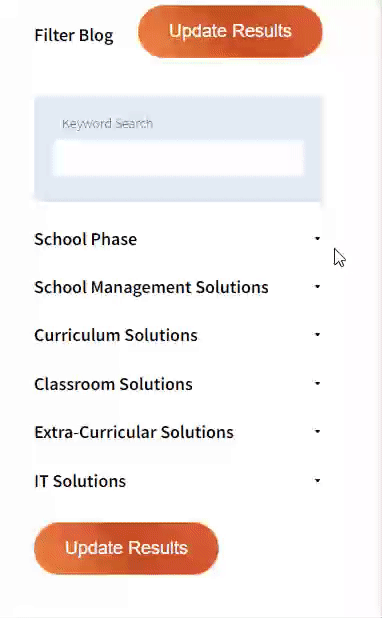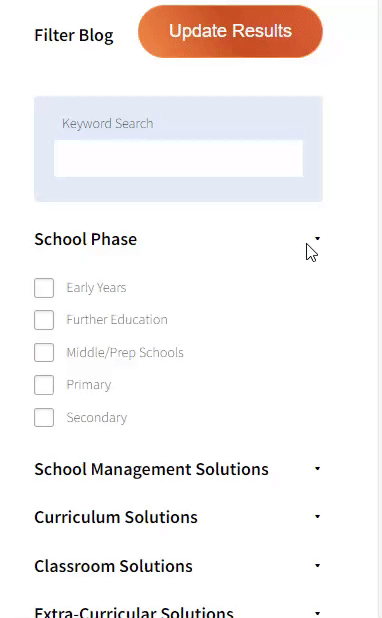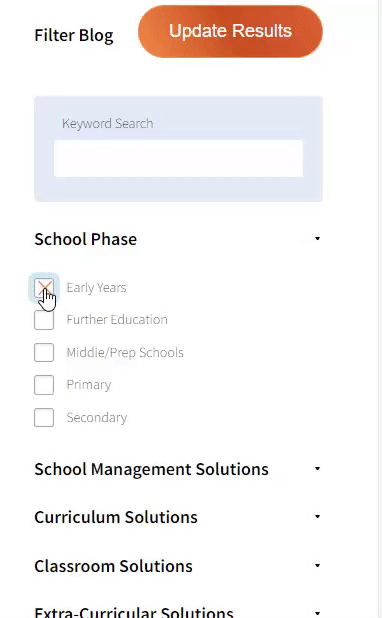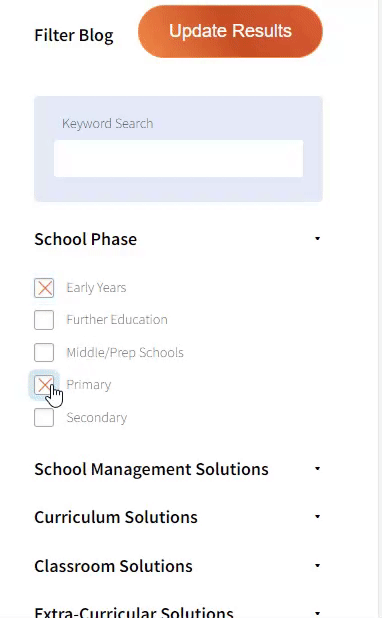
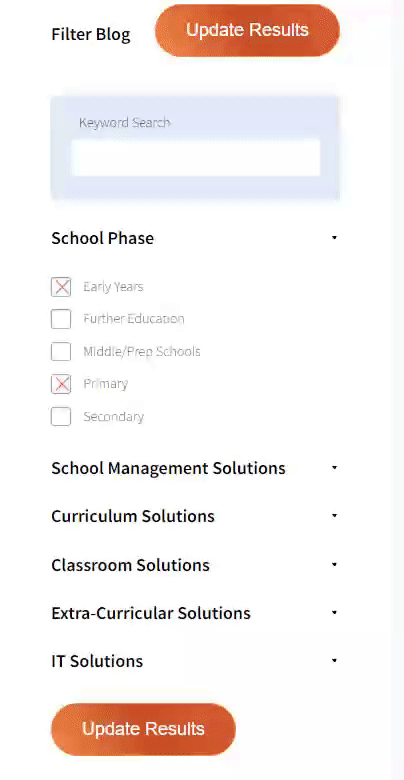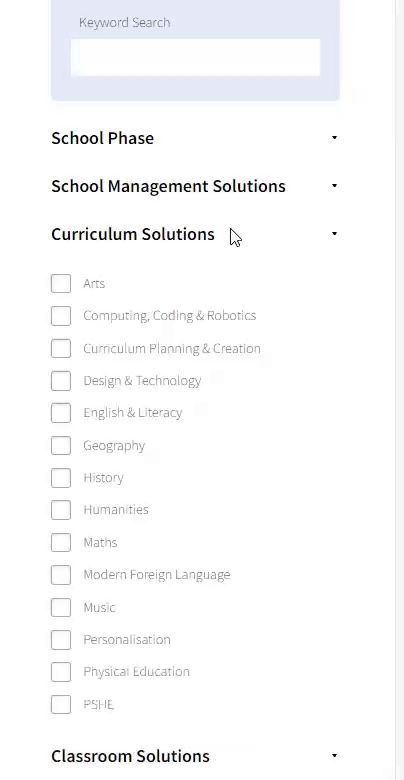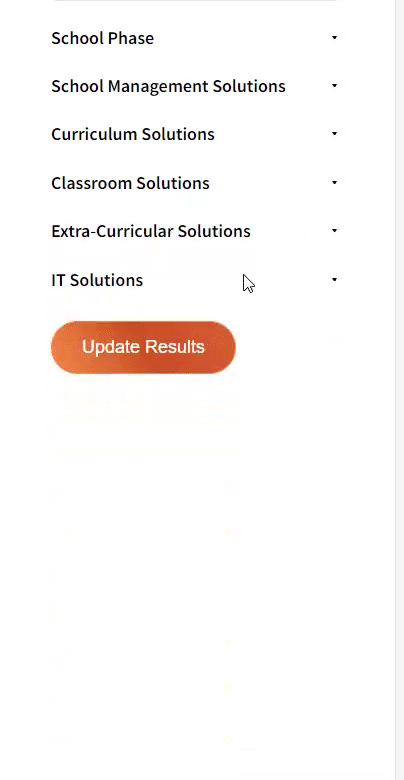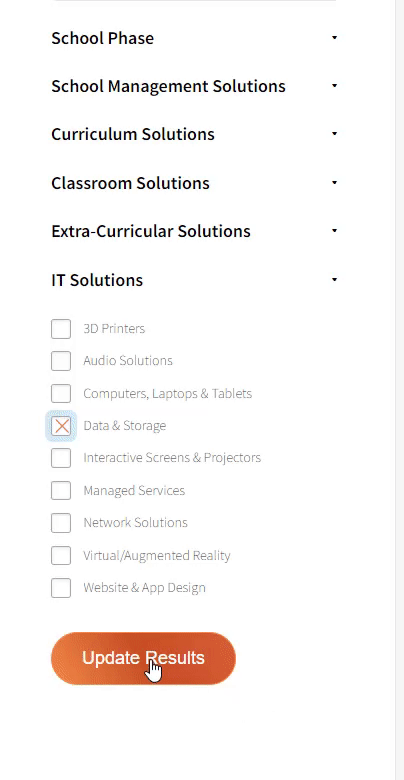
And there you have it! Now your collection of blogs are catered to your chosen topics and are ready for you to explore. Plus, if you frequently return to the same categories you can bookmark your current URL and we will save your choices on return. Happy Reading!
Giving children a reason to use technology. I’ve been striving to make sure that the children have real connected purposes for learning in our classroom this year, as linking as many skills from as many subjects in one project is so very important. I feel this is essential for learning in every classroom, whether in Primary or Secondary schools.
Children need to see the point of learning these skills – “what are people using these skills for right now in the world and what can I use them for?”
Thinking more about the outcome and then working backwards to find the skills needed, is something we need to do more in our schools. Don’t ruin the surprise for the children; allow the learning to be a surprise.
Don’t start with a learning objective, start with a question and see where it leads.
I started the year with the children getting enthusiastic on Edmodo by asking them to suggest ways in which we could use Minecraft in school. One child, in particular, had a great idea to test various blocks on Minecraft using a diamond pickaxe – if you’re not sure about what a diamond pickaxe is, ask any of your children in school. They are the experts, and it’s important to remember this when technology comes into schools. Just ask them.

From the image, you can clearly see how organised his thinking is and even when he was pushed you could see the scientific methods he’d been taught coming to the forefront. All of this pre-planning had taken place before the school year had even started.
It was great watching him carry out his investigation in class as he’d planned it and was so very excited to do it. It was pleasing to see how he’d thought it through and planned to use his findings to help him in the game. We predicted that the diamond pickaxe was going to be the quickest, but I asked him for evidence and he provided it by following a scientific investigation.
He used the techniques we’d learned in school to plan and carry out his investigation. A true test of the application of skills. To add to this he even published his results using a bar graph – a good use of his maths skills. I’ve no idea why he chose 28 cobblestone blocks, but crucially he kept it fair. Added to this, he was using a stopwatch on his iPad to time the experiment to two decimal places, “just in case it’s really close.” He is planning other experiments.

Using Minecraft allowed him to carry out a real-life investigation using the skills he’d learned to solve a problem that he was interested in. He wanted to find out once and for all which was the best pickaxe. We now have the scientific proof that a diamond pickaxe is better at mining cobblestone in Minecraft.
Analysing the results started to raise more investigation questions, just like any good investigation should. He was curious to find out if other mining tools had a similar pattern or if these results would display similar results.
Area and Perimeter: Using Minecraft
We started to investigate Area and Perimeter in mathematics, built on the idea that architects need to use this information to build our houses and schools. The children wanted to know how buildings were constructed and how their homes had been designed. What did those designs look like?
Building on from this question, we started to think about why kitchens are one size, but bedrooms and gardens are another size. Using Minecraft and the idea that a bed takes two blocks, we could start planning room sizes in creative mode.
We used the blocks to calculate the area of the rooms we built by counting the blocks, a simple but effective way to calculate the area of a shape. We then quickly made the leap into making our calculations more efficient by thinking about how arrays worked and how we could multiply the length by the width to find the area.
We moved on to splitting irregular shapes into two or three shapes and used different coloured blocks to calculate. During these sessions, the children discovered the formula for calculating the area and it spread around the classroom.
The children then took this ‘playing’ into their maths books and demonstrated a clear understanding of how to find the area of regular and irregular shapes following a formula they’d discovered.

The author

Read more

Read more

Read more

Read more

Read more

Read more

Read more

Read more


Are you looking for solutions? Let us help fund them! Nexus Education is a community of over 11,000 schools that come together to share best practise, ideas and CPD via online channels and free to attend events. Nexus also offers funding to all school groups in the UK via nexus-education.com


Established in 2011, One Education is a company at the heart of the education world, supporting over 600 schools and academies. Our unique appeal as a provider is in the breadth and synergy of the services we offer, supporting school leaders, teachers and support staff to achieve the best possible outcomes for their pupils and staff.

School Space is a social enterprise that has empowered schools for over 12 years through their profitable and hassle-free lettings services. So far, they’ve generated over £5 million in revenue for education, helping to connect over 200 schools with their local communities.


Unify is an online sales and marketing tool that allows users to create tailored personalised documents in moments.


There’s nothing special about the energy we sell. In fact, it’s exactly the same energy as all our competitors provide. But there is something special about the way we do it. Where others complicate the process, we simplify it. Where others confuse customers with hidden terms, we’re an open book. And where others do all they can to make as much money from their customers as possible, we do all we can to make as little. Everything we do, we do it differently. Our customers are a privilege. One we’ll never take advantage of.


Securus provide market-leading monitoring solutions to safeguard students on ALL devices both online and offline. We also offer a full monitoring service, where we carry out the monitoring on behalf of the school, freeing up valuable staff resources. From the smallest school to large MAT groups, Securus offers safeguarding protection for all!


Bodet Time offers dedicated solutions to education through lockdown alerts, class change systems, PA and synchronised clock systems. Improving time efficiency of the working and school day; ensuring safety through lockdown alerts; increasing communication with customised broadcast alerts.


Robotical makes Marty the Robot - a walking, dancing coding robot that makes programming fun and engaging for learners as young as 5. Our robots come with a full Learning Platform that has complete teaching resources, to make lesson planning a breeze.